This exercise is from Nonlinear Dynamics and Chaos, 2nd Edition by Steven H. Strogatz

• Part 1: One-Dimensional Flows

  • 2 Flows on the Line

Exercise 2.2.3
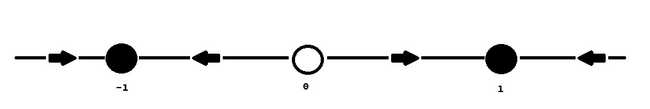
Analyze the following equation graphically. Sketch the vector field on the real line, find all the fixed points, classify their stability, and sketch the graph of $x(t)$ for different initial conditions. Then try to obtain the analytical solution for $x(t)$.

$\dot {x} = x - x^{3}$

From the above graph of $\dot {x} = x - x^{3}$, it's straightforward to sketch the vector field shown below it. The fixed points are $x*$ = -1, 0, and 1. $\dot {x}$ is positive to the left of $x*$ = -1, so the flow is to the right. To the right of $x*$ = -1, $\dot {x}$ is negative, so the flow is to the left. From these two facts, it's clear that $x*$ = -1 is a stable fixed point. Using the same analysis for the other fixed points, we conclude that that $x*$ = 0 is an unstable fixed point and $x*$ = 1 is a stable fixed point.

An analytic solution is possible.

$\dfrac{\mathrm{d} x}{\mathrm{d} t} = x - x^{3} = x(1 -x^{2}) = x(1+x)(1-x)$

$\dfrac{1}{x(1+x)(1-x)}dx = dt$

$\displaystyle\int \dfrac{1}{x}dx - \dfrac{1}{2}\int \dfrac{1}{x+1}dx - \dfrac{1}{2}\int\dfrac{1}{x-1}dx = \int_{0}^{t}dt$

$ln\left |x \right | - \dfrac{1}{2}ln\left |x+1 \right | - \dfrac{1}{2}ln\left |x-1\right | + C = t$

$\pm \dfrac{x^{2}}{x^{2} - 1} = ke^{2t}$ (must consider + and - solutions)

$x(t) = \pm \dfrac{\sqrt{k}e^{t}}{\sqrt{ke^{2t}-1}}$

and

$x(t) = \pm \dfrac{\sqrt{k}e^{t}}{\sqrt{ke^{2t}+1}}$

Solutions tend toward $\pm \ 1$ as t goes to $\pm \infty$